

The sound of gravitational waves from a [confinement] phase transition
saoghal.net/slides/ectstar/
David J. Weir - University of Helsinki - davidjamesweir
Interdisciplinary approach to QCD-like composite dark
matter
ECT*, 4.10.2018
What happened during the electroweak phase transition? when the universe was optically opaque? in dark sectors?
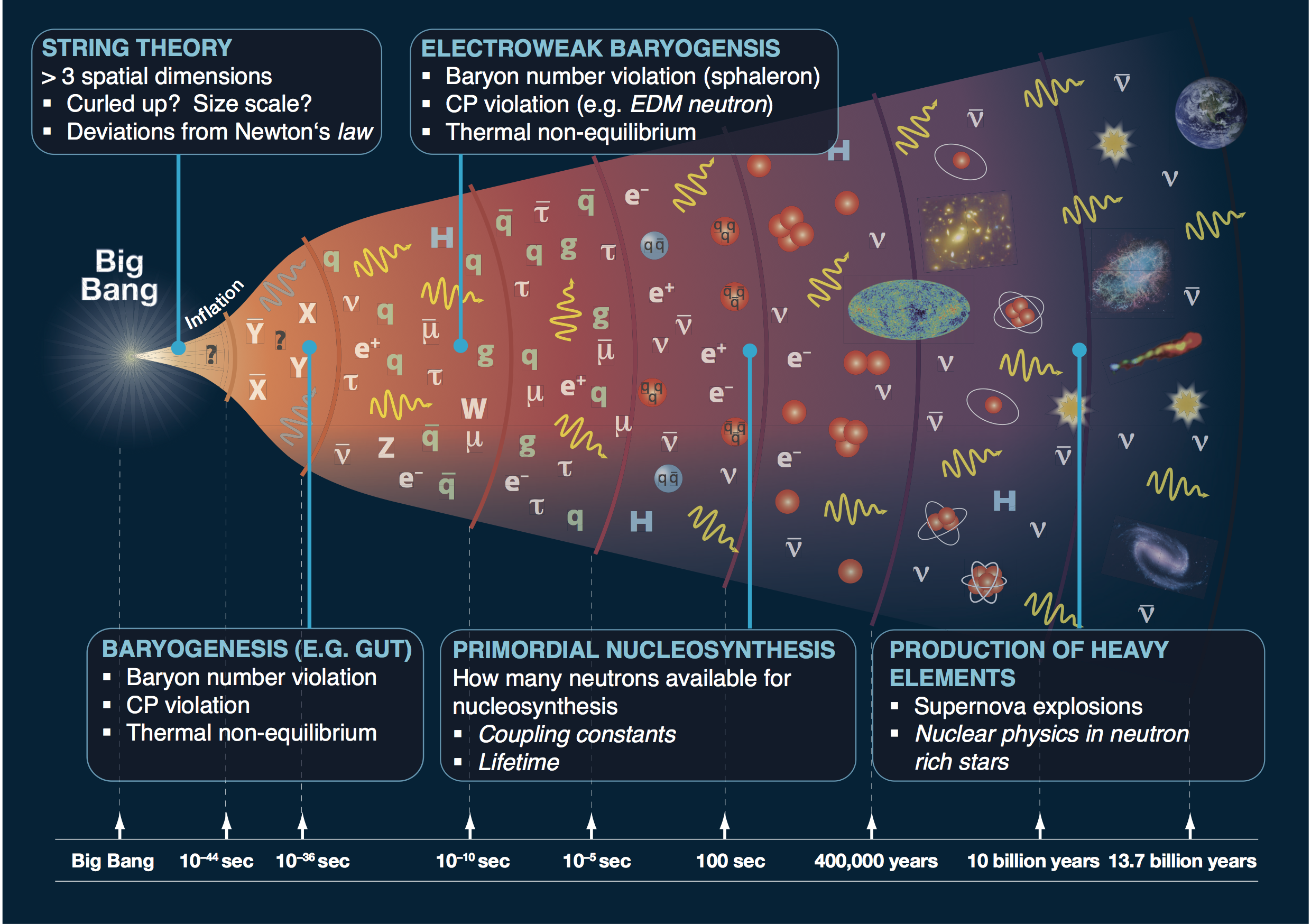
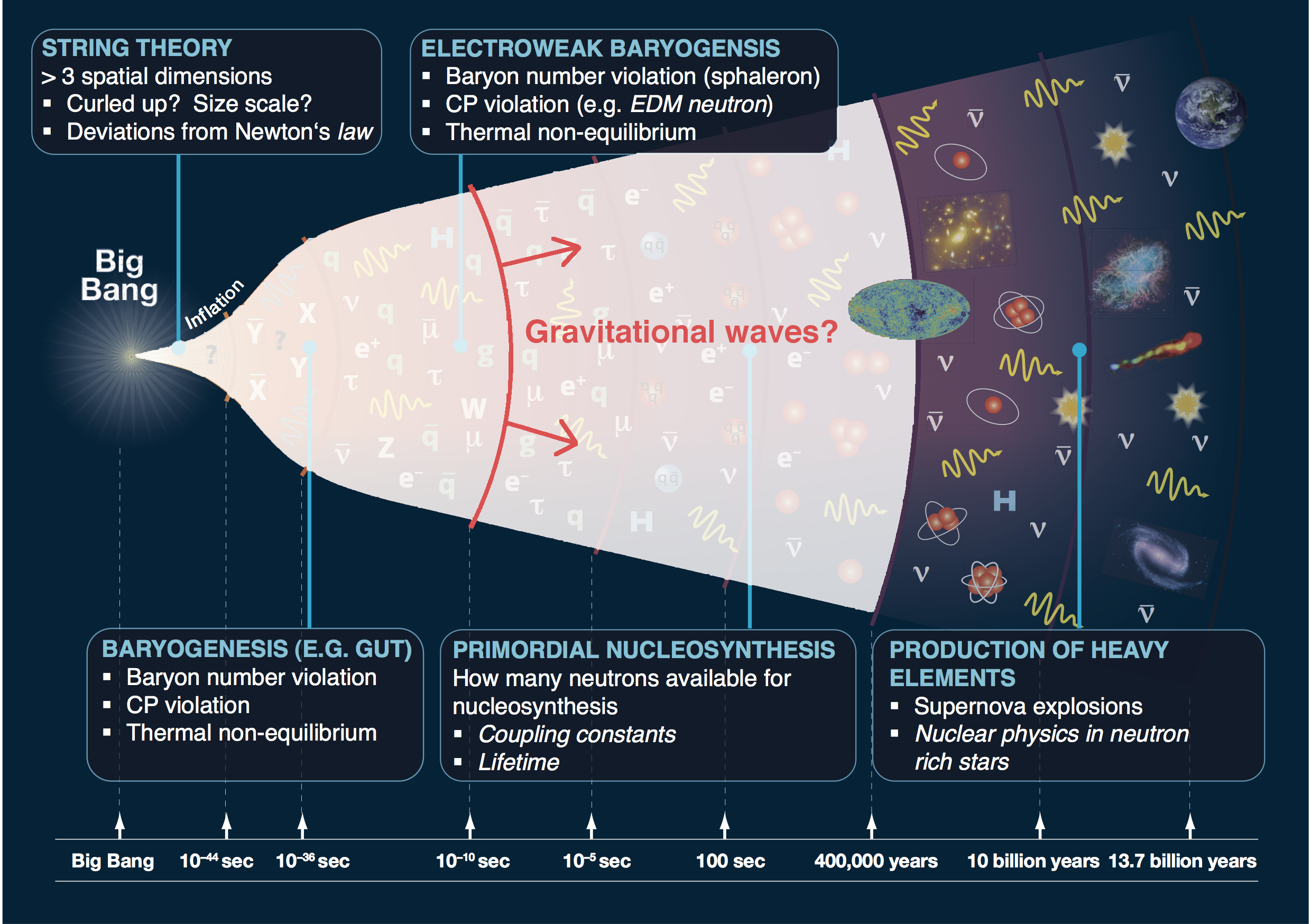

What's next: LISA mission

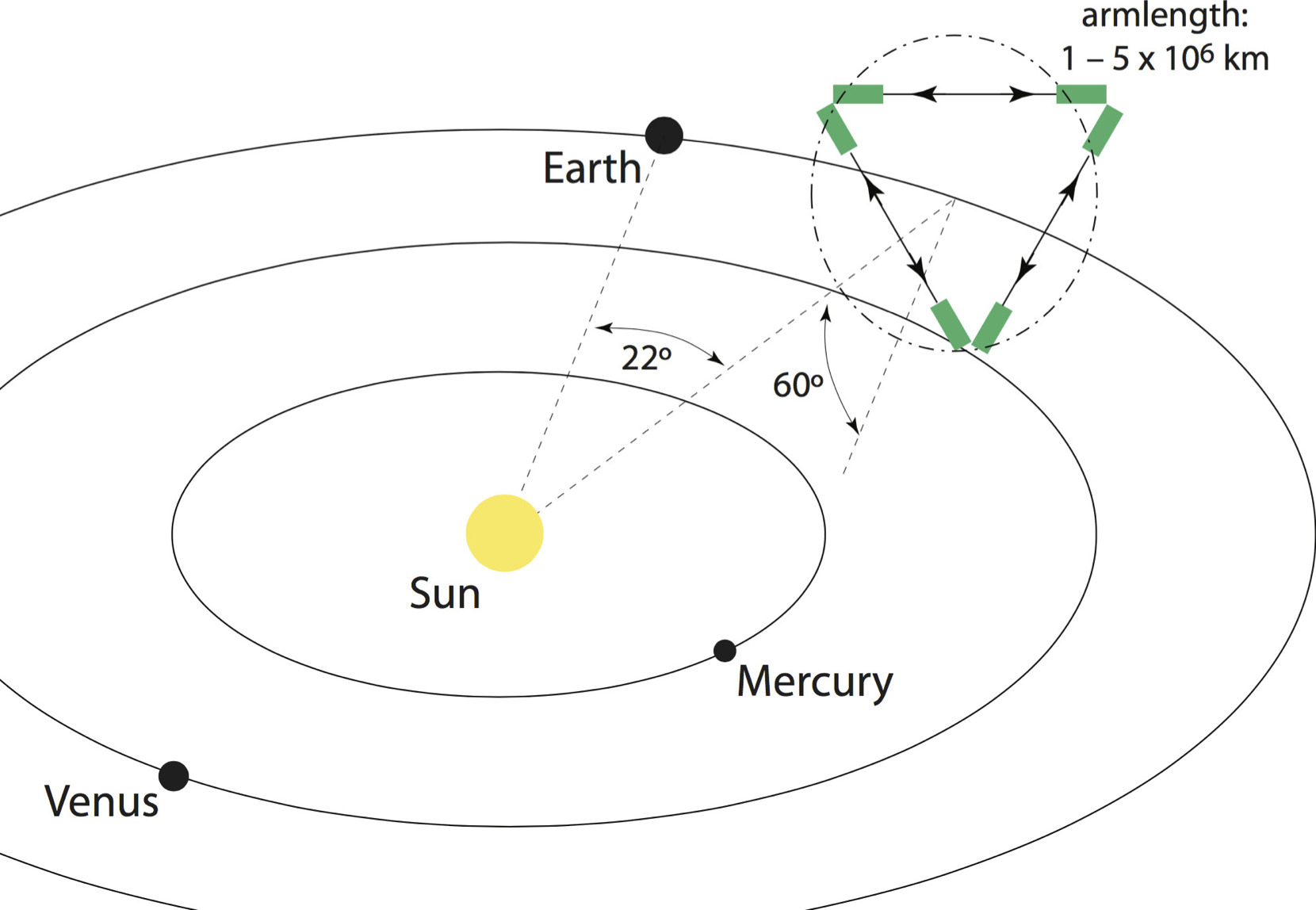
- Three laser arms, 2.5 M km separation
- ESA-NASA mission, launch by 2034
- Proposal submitted last year arXiv:1702.00786
- Officially adopted on 20.6.2017
LISA Pathfinder
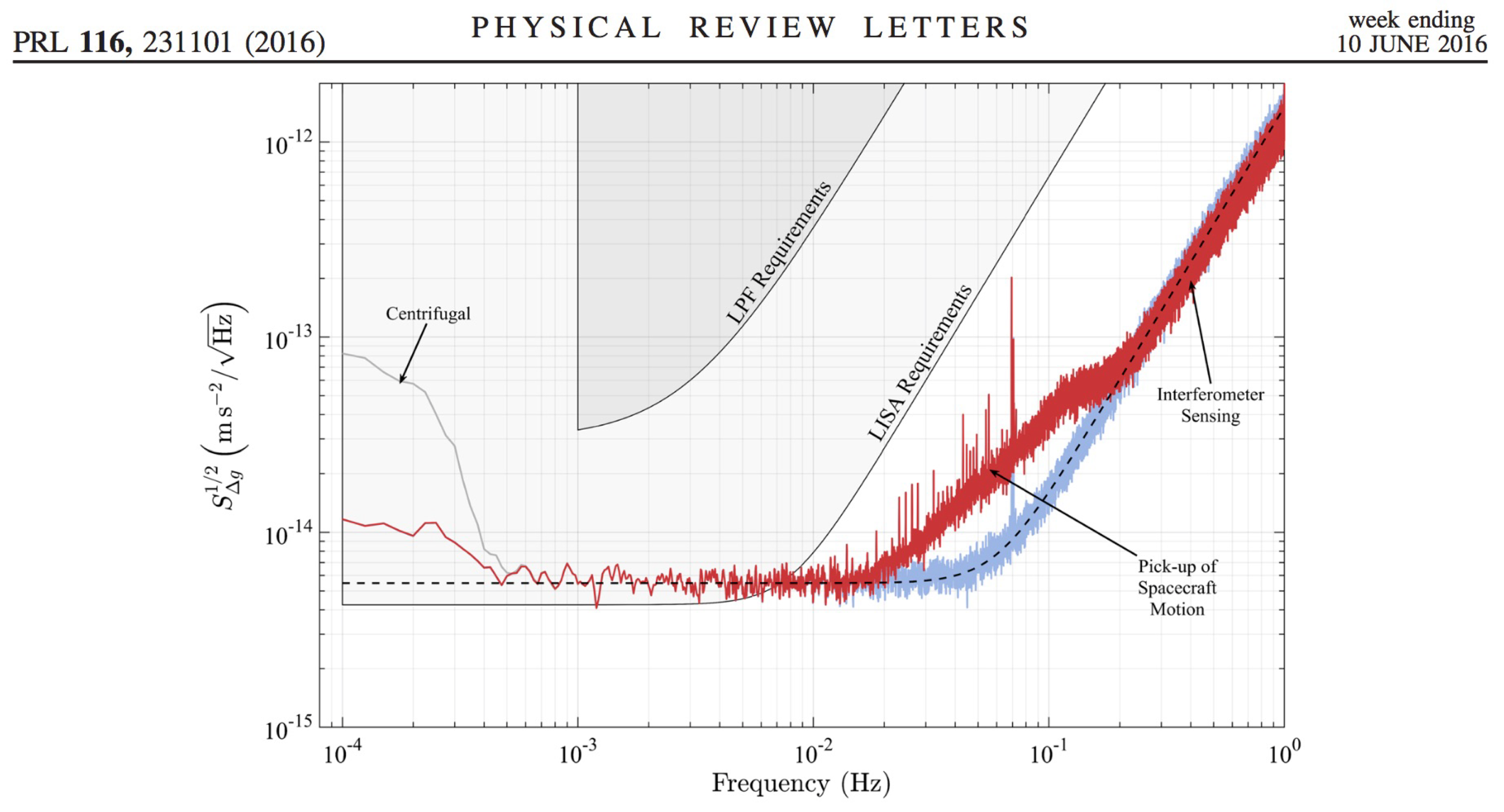
Exceeded design expectations by factor of five!
Possible signals
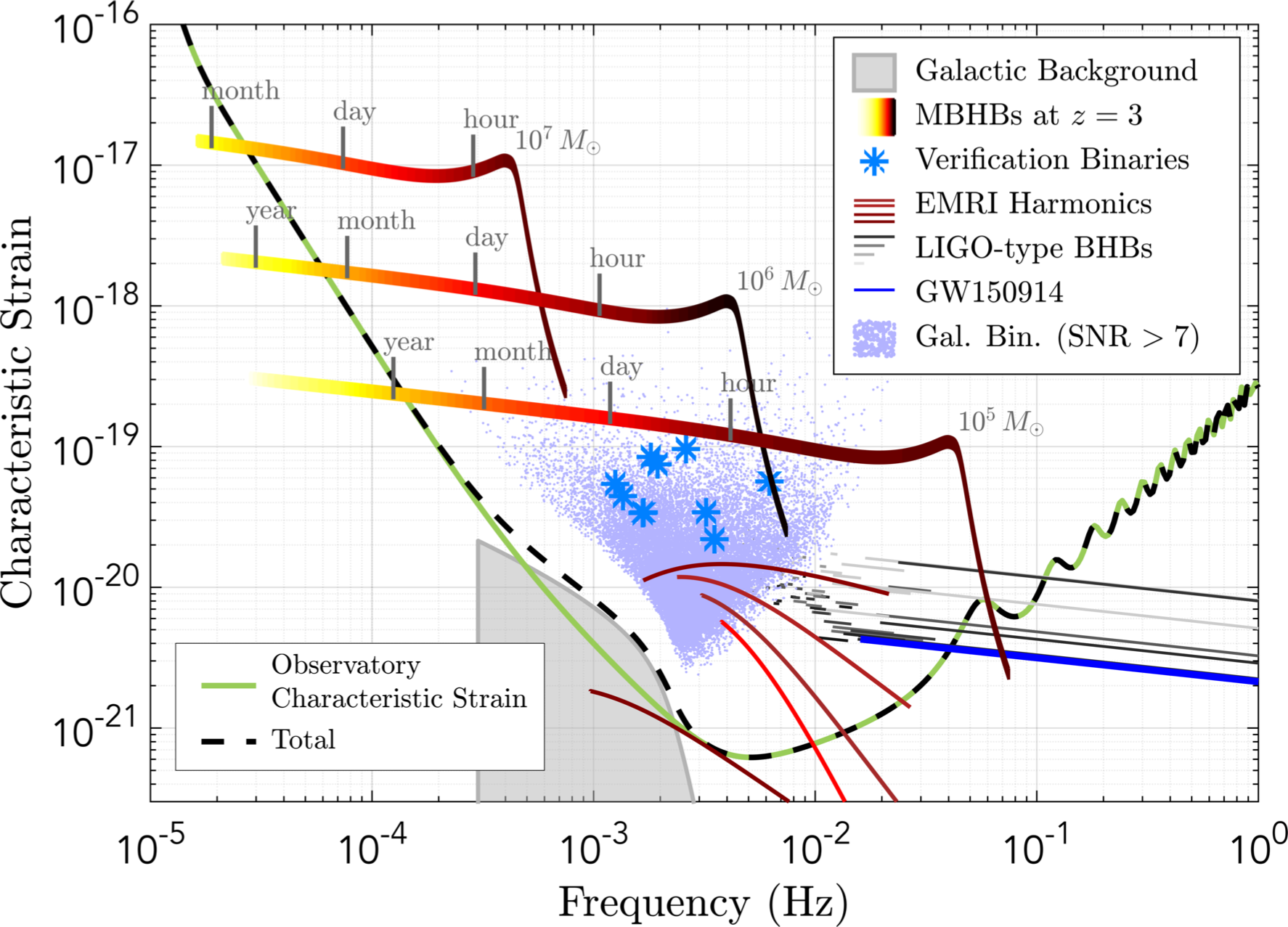
Source: arXiv:1702.00786.
Key science for LISA

Science Investigation 7.2: Measure, or set upper limits on, the spectral shape of the cosmological stochastic GW background.
Operational Requirement 7.2: Probe a broken power-law stochastic background from the early Universe as predicted, for example, by first order phase transitions ...
First order thermal phase transition
- Bubbles nucleate and grow
- Expand in a plasma - create reaction fronts
- Bubbles + fronts collide - violent process
- Sound waves left behind in plasma
- Turbulence; damping
Key parameters for GW production
4 numbers parametrise the transition:
- $T_*$, temperature ($\approx T_\mathrm{n} \lesssim T_\mathrm{c}$)
- $\alpha_{T_*}$, vacuum energy fraction
- $v_\mathrm{w}$, bubble wall speed
- $\beta/H_*$:
- $\beta$, inverse phase transition duration
- $H_*$, Hubble rate at transition
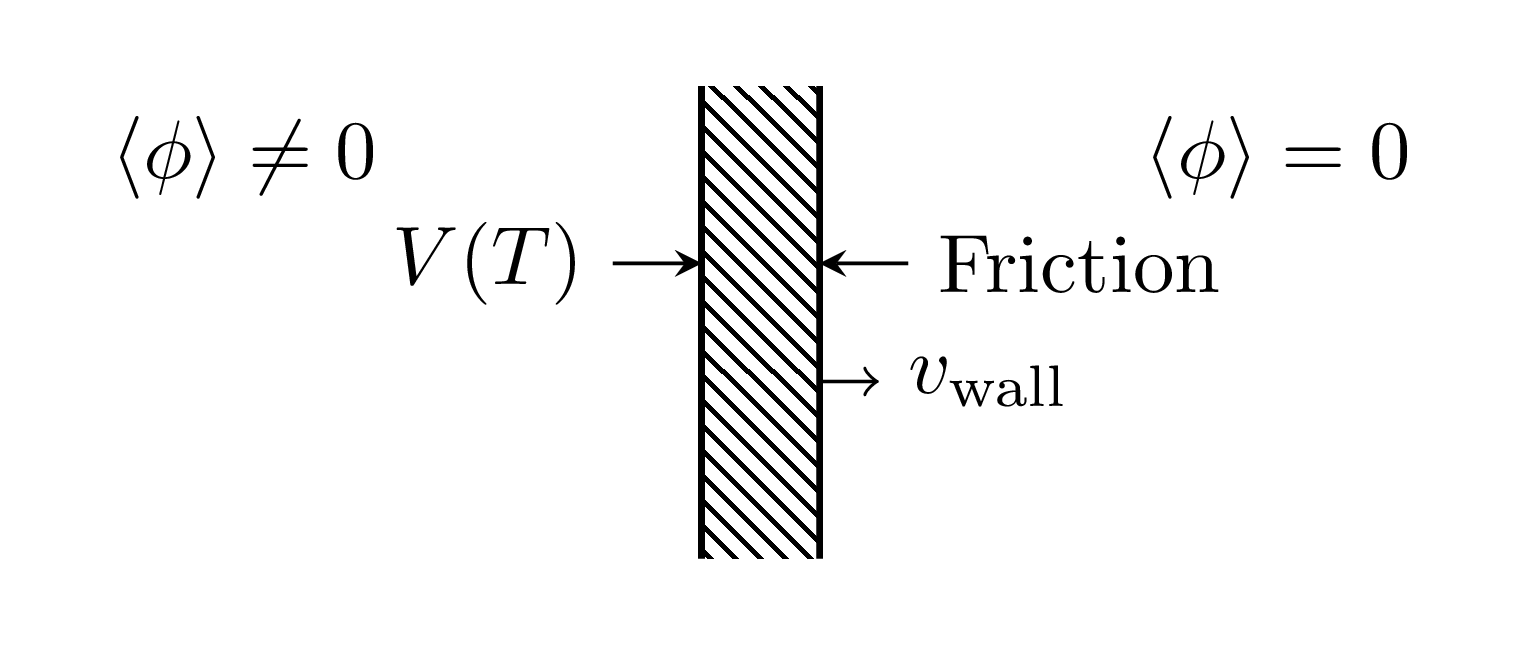
How the wall moves
- In EWPT: equation of motion is (schematically)
Liu, McLerran and Turok; Prokopec and Moore; Konstandin, Nardini and Rues; ... $$ \partial_\mu \partial^\mu \phi + V_\text{eff}'(\phi,T) + \sum_{i} \frac{d m_i^2}{d \phi} \int \frac{\mathrm{d}^3 k}{(2\pi)^3 \, 2 E_i} \delta f_i(\mathbf{k},\mathbf{x}) = 0$$- $V_\text{eff}'(\phi)$: gradient of finite-$T$ effective potential
- $\delta f_i(\mathbf{k},\mathbf{x})$: deviation from equilibrium phase space density of $i$th species
- $m_i$: effective mass of $i$th species:
Force interpretation
$$ \overbrace{\partial_\mu T^{\mu\nu}}^\text{Force on $\phi$} - \overbrace{\int \frac{d^3 k}{(2\pi)^3} f(\mathbf{k}) F^\nu }^\text{Force on particles}= 0 $$This equation is the realisation of this idea:
Fluid interpretation
Yet another interpretation:
$$ \overbrace{\partial_\mu T^{\mu\nu}}^\text{Field part} - \overbrace{\int \frac{d^3 k}{(2\pi)^3} f(\mathbf{k}) F^\nu }^\text{Fluid part}= 0 $$i.e.:
$$ \partial_\mu T^{\mu\nu}_\phi + \partial_\mu T^{\mu\nu}_\text{fluid} = 0 $$Can simulate as effective model of field $\phi$ + fluid $u^\mu$.
What the makes the GWs at a thermal phase transition?
- Bubbles nucleate and expand, shocks form, then:
- $h^2 \Omega_\phi$: Bubbles + shocks collide - 'envelope phase'
- $h^2 \Omega_\text{sw}$: Sound waves set up - 'acoustic phase'
- $h^2 \Omega_\text{turb}$: [MHD] turbulence - 'turbulent phase'
- Sources add together to give observed GW power: $$ h^2 \Omega_\text{GW} \approx h^2 \Omega_\phi + h^2 \Omega_\text{sw} + h^2 \Omega_\text{turb}$$
Step 1: Envelope phase?
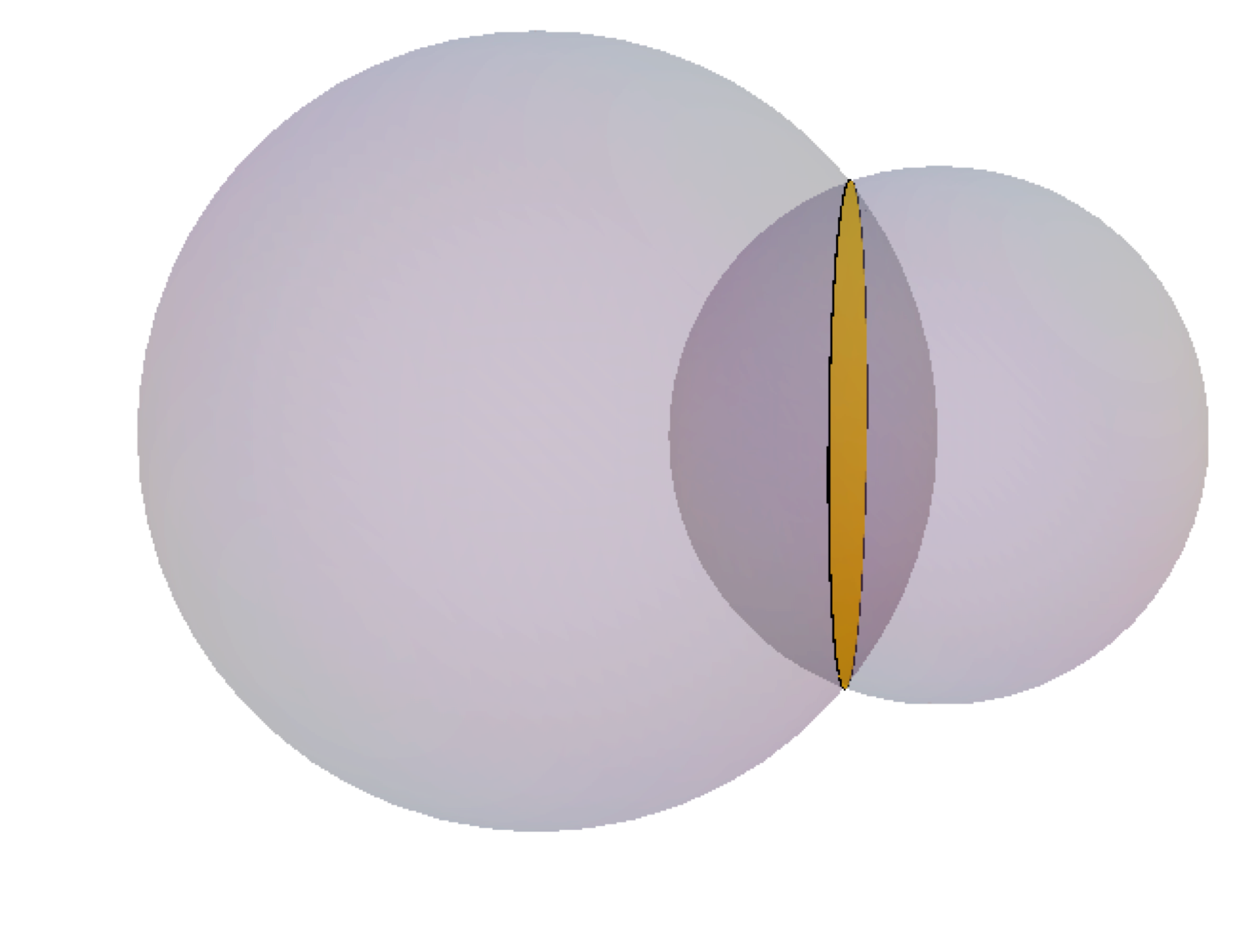
Hope: envelope approximation
- Finite $v_\mathrm{w}$
- Thin, hollow bubbles, no fluid
- Stress-energy tensor $\propto R^3$ on wall
- Solid angle: overlapping bubbles → GWs
- Simple power spectrum:
- One length scale (average radius $R_*$)
- Two power laws ($\omega^3$, $ \sim \omega^{-1}$)
- Amplitude
⇒ 4 numbers define spectral form
Hope: Envelope approximation
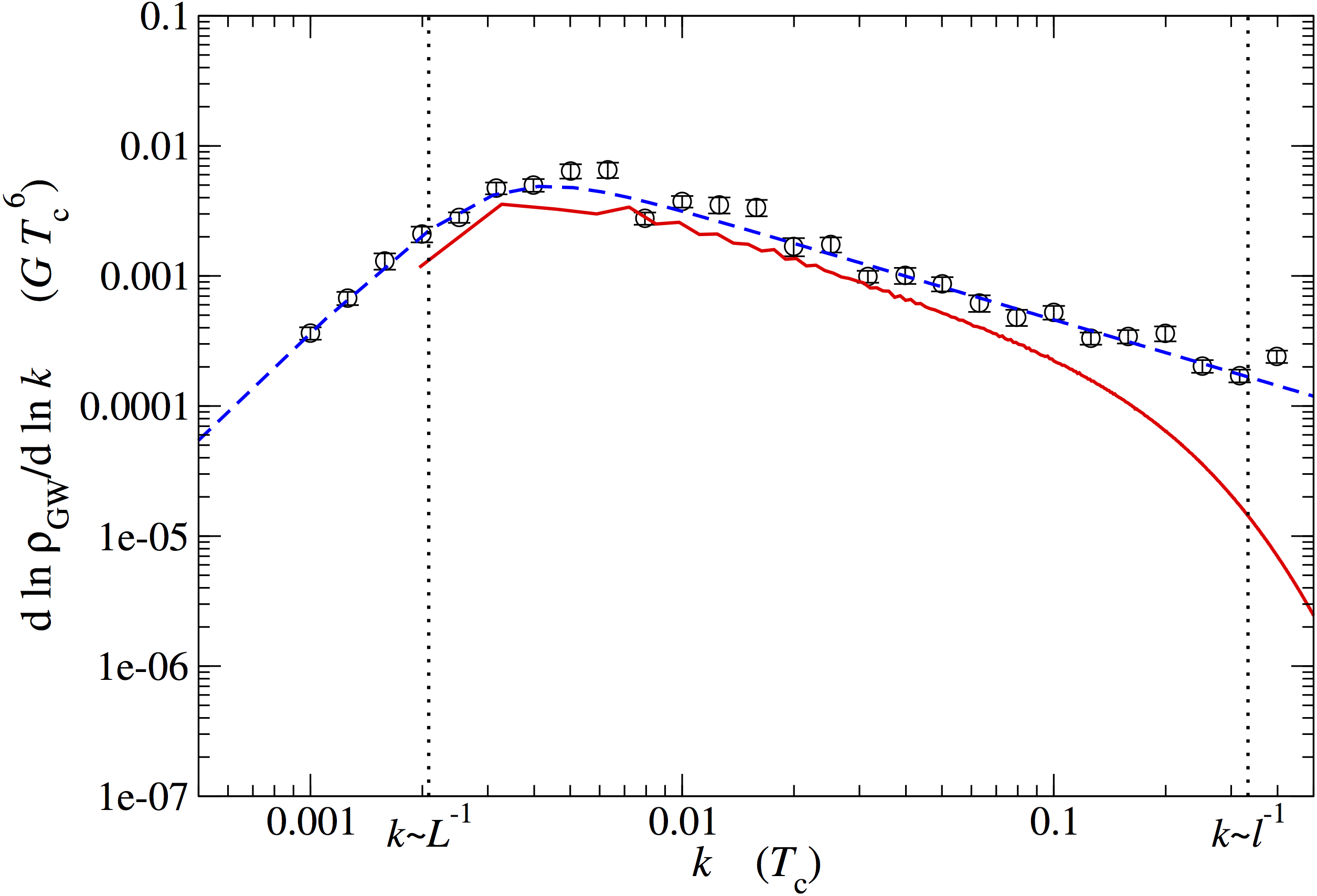
Source: arXiv:1604.08429
When $v_\mathrm{w}$ finite, nice $k^{-1}$ power law - agrees with lattice
Reality: Vacuum transitions (large $v_\mathrm{w}$)
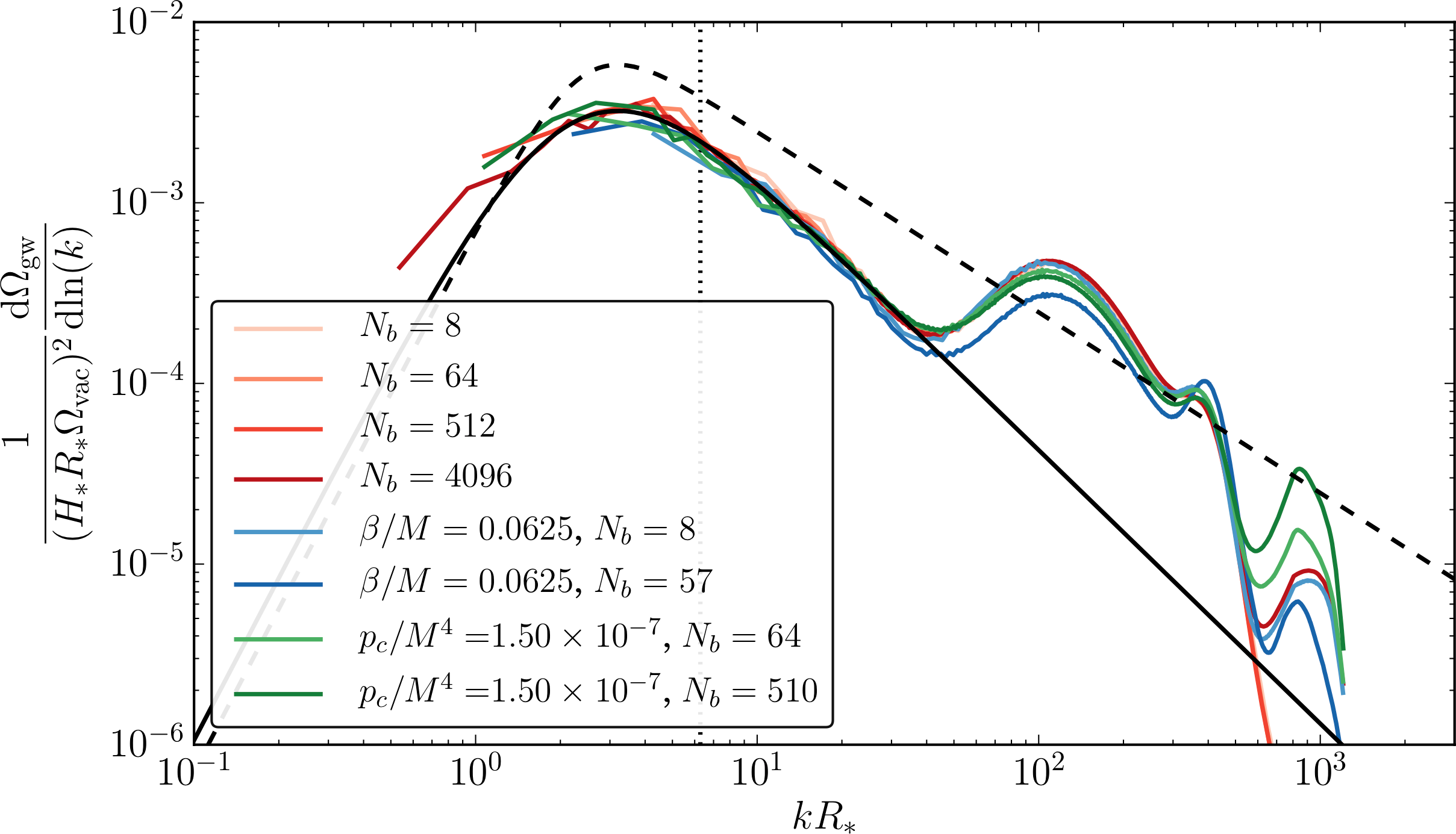
Source: arXiv:1802.05712
When $v_\mathrm{w}$ ultrarelativistic, power law steeper
Reality: Envelope approximation
- For most transitions: most energy in plasma
- Ultra-relativistic: disagrees with envelope approximation
Claim: Envelope approximation can be safely ignored
Step 1: Envelope phase?
Step 2: Acoustic phase
Coupled field and fluid system
astro-ph/9309059- Scalar $\phi$ and ideal fluid $u^\mu$:
- Split stress-energy tensor $T^{\mu\nu}$ into field and fluid bits $$\partial_\mu T^{\mu\nu} = \partial_\mu (T^{\mu\nu}_\phi + T^{\mu\nu}_\text{fluid}) = 0$$
- Parameter $\eta$ sets the scale of friction due to plasma $$\partial_\mu T^{\mu\nu}_\phi = \tilde \eta \frac{\phi^2}{T} u^\mu \partial_\mu \phi \partial^\nu \phi \quad \partial_\mu T^{\mu\nu}_\text{fluid} = - \tilde \eta \frac{\phi^2}{T} u^\mu \partial_\mu \phi \partial^\nu \phi $$
- $V(\phi,T)$ is a 'toy' potential tuned to give $\alpha_{T_*}$
- $\beta/H_*$ ↔ number of bubbles, $v_\text{wall}$ ↔ $\tilde \eta$
What sort of solutions does this system have?
Reaction front
- At a reaction front: chemical transformation. Chemically and physically different on each side.
- Different from a shock front, where the energy density and entropy change.
- We have a reaction front as $\langle \phi \rangle = 0$ before and $\langle \phi \rangle \neq 0$ after.
Detonations vs deflagrations
- If $\phi$ wall moves supersonically and the
fluid $u^\mu$ enters the wall at rest, we have
a detonation
☛ Generally good for GWs - If $\phi$ wall moves subsonically and the
fluid $u^\mu$ enters the wall at its maximum velocity, it's
a deflagration
☛ Generally bad for GWs
Velocity profile development: detonation vs deflagration
Simulation slice example
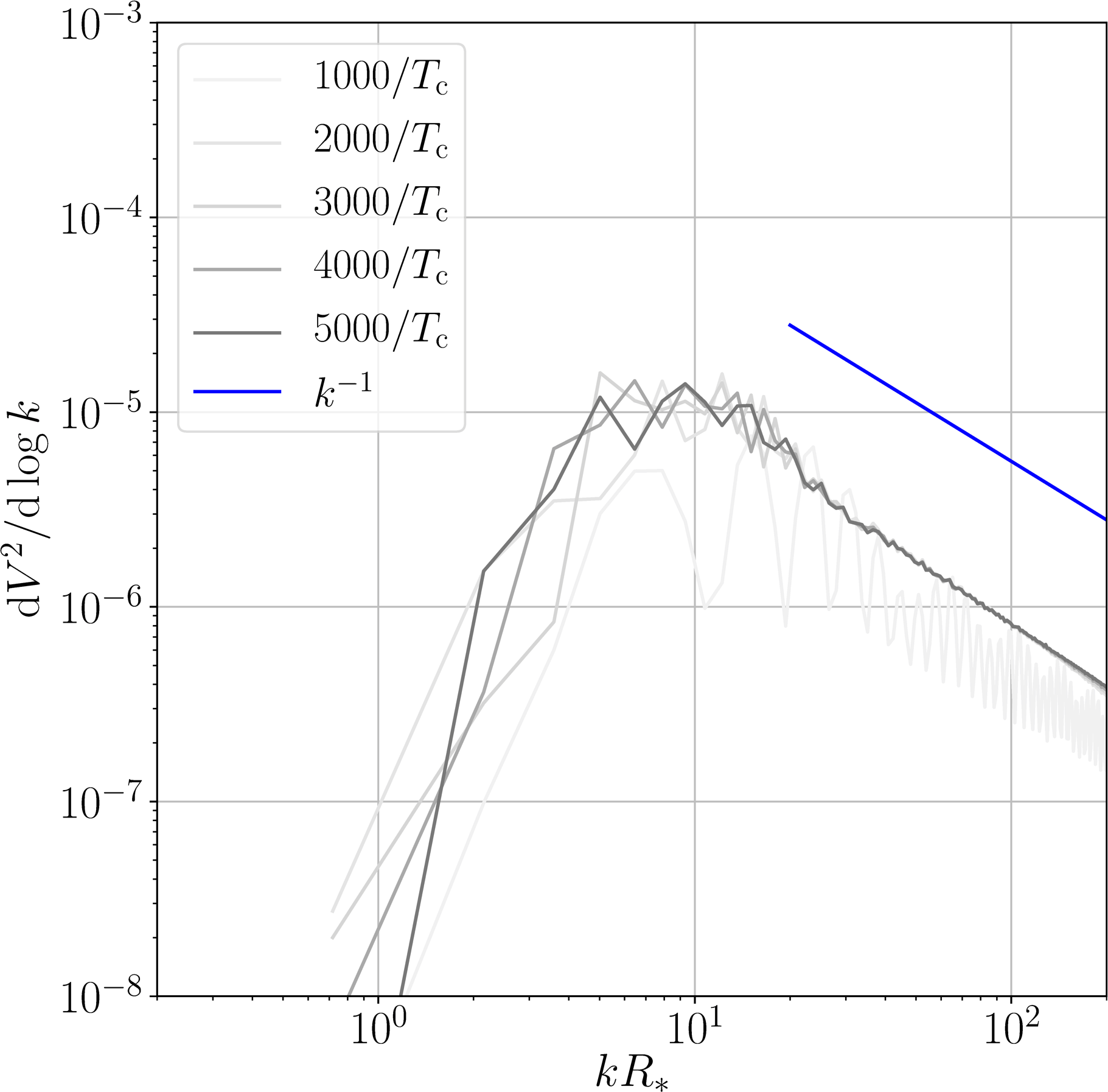
Velocity power spectra
$v_\mathrm{w} < c_\mathrm{s}$
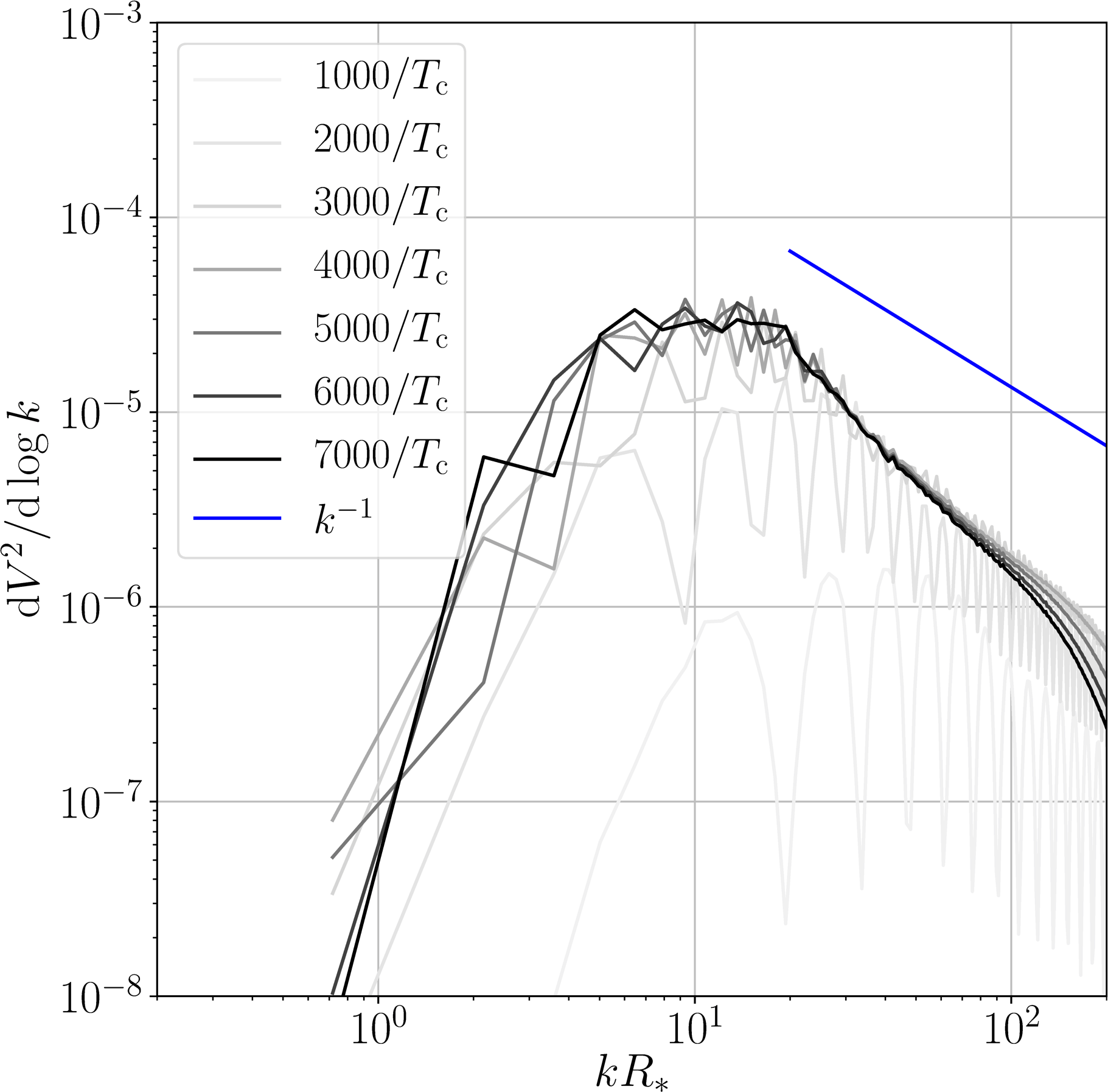
$v_\mathrm{w} > c_\mathrm{s}$
Computing $\Omega_\text{GW}$
- Simply evolve numerically: $$ \square \, h_{ij}(x,t) = 16 \pi G \, T_{ij}^\text{source}(x,t). $$
- When $T_{ij}^\text{source} =
(\epsilon + p) u_i u_j$ ➸ convolution of
fluid velocity
arXiv:0909.0622 - To measure the energy in gravitational waves, project out transverse-traceless part: $$ t_{\mu\nu}^\text{GW}= \frac{1}{32 \pi G}\langle \partial_\mu h^\text{TT}_{ij} \partial_\nu h^\text{TT}_{ij} \rangle; \quad \rho_\text{GW} = \frac{1}{32 \pi G} \langle \dot{h}^\text{TT}_{ij} \dot{h}^\text{TT}_{ij} \rangle. $$
- We can then redshift this to present day to get $\Omega_\text{GW} h^2$.
GW power spectra and power laws
$v_\mathrm{w} < c_\mathrm{s}$
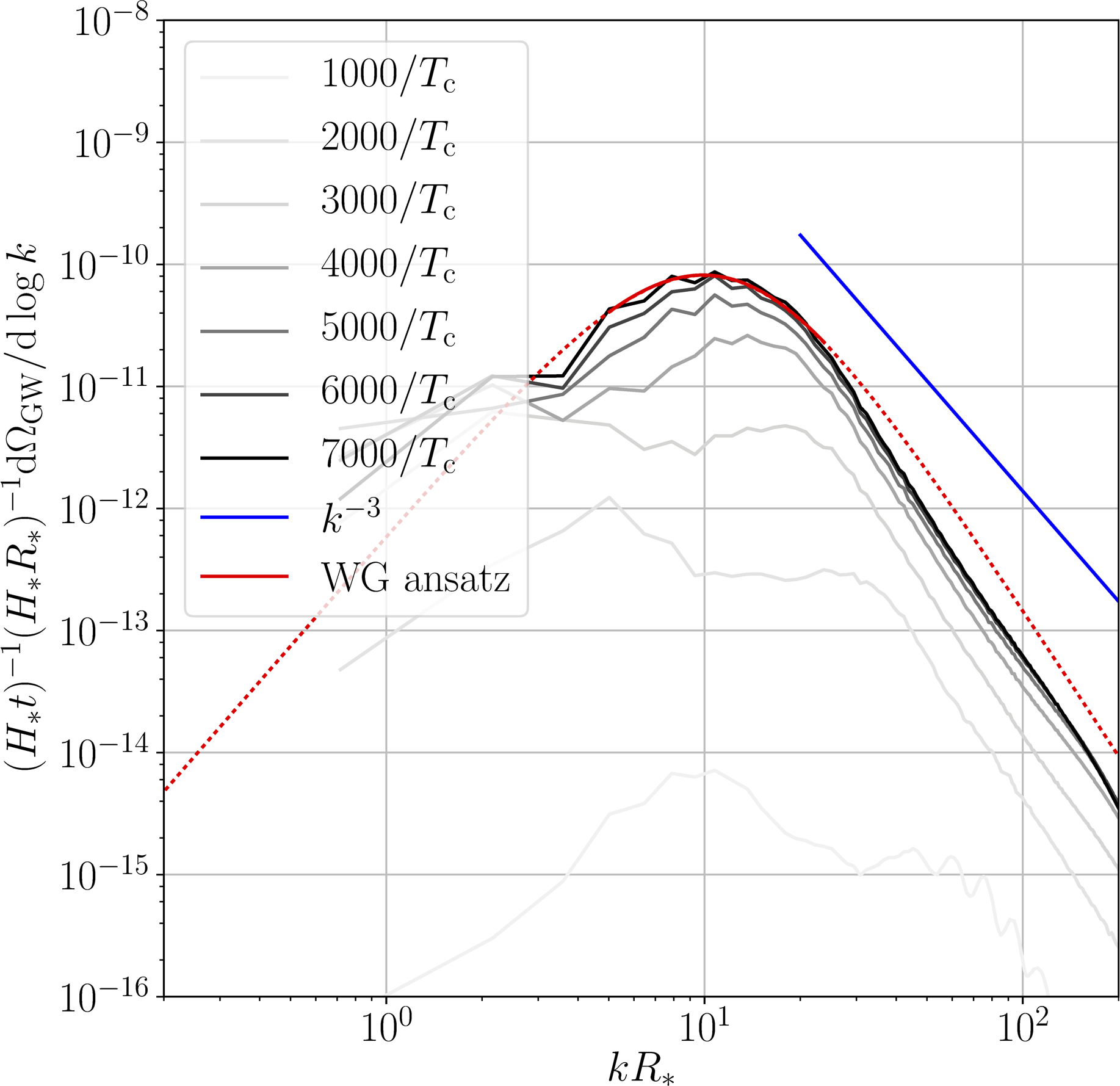
$v_\mathrm{w} > c_\mathrm{s}$

NB: curves scaled by $t$
Step 1: Envelope phase?
Step 2: Acoustic phase 👍
Step 3: Turbulence 🤔

Source: Wikimedia Commons/Gary Settles (CC-BY-SA)
Shocks and turbulence?
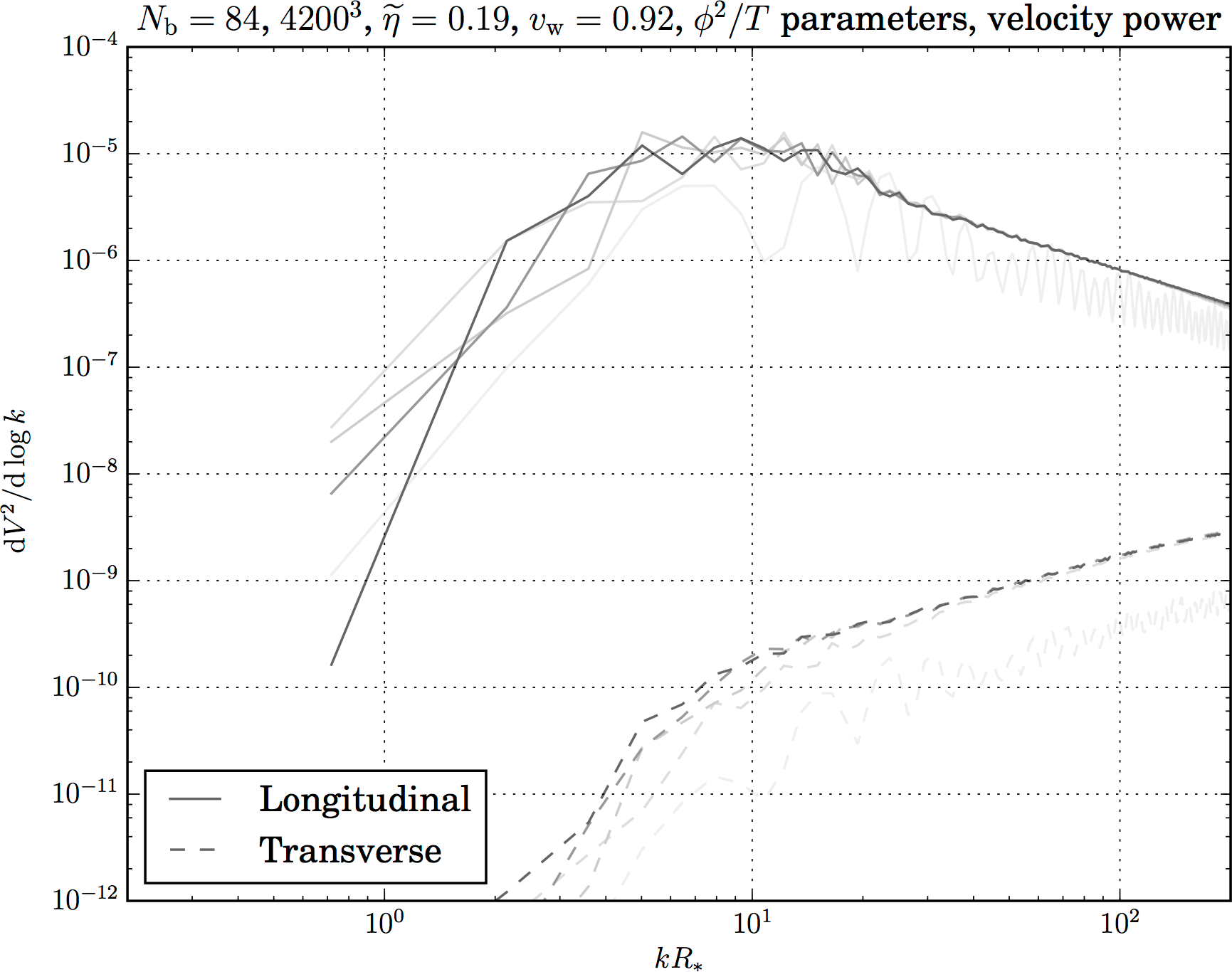
- Require longer timescales (fluid turnover time)
$R_*/\overline{U}_\mathrm{f}$,
thus: may not develop at all - Plenty of theoretical results, but little agreement
arXiv:0705.1733; arXiv:0909.0622; arXiv:1510.02985; ...
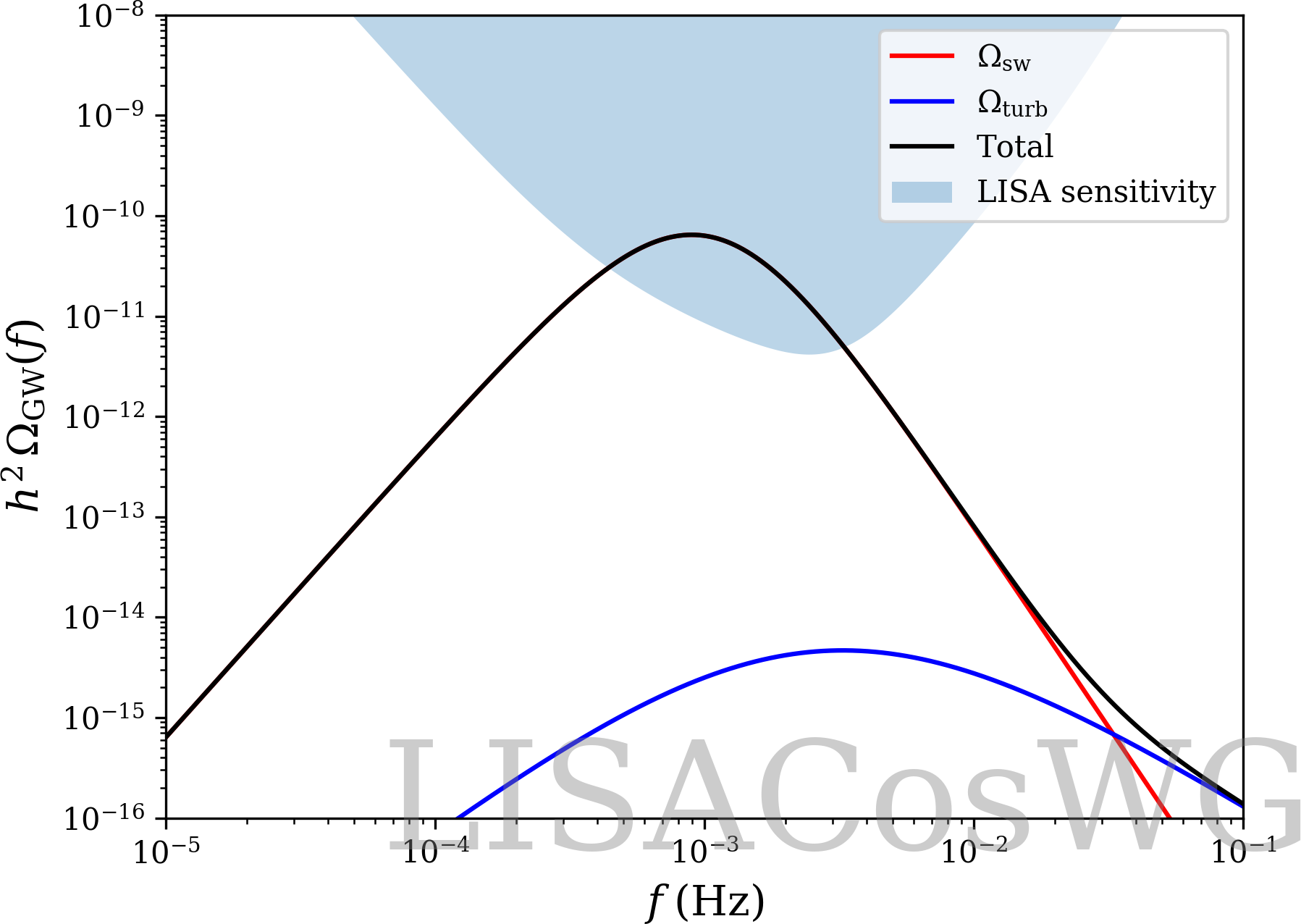
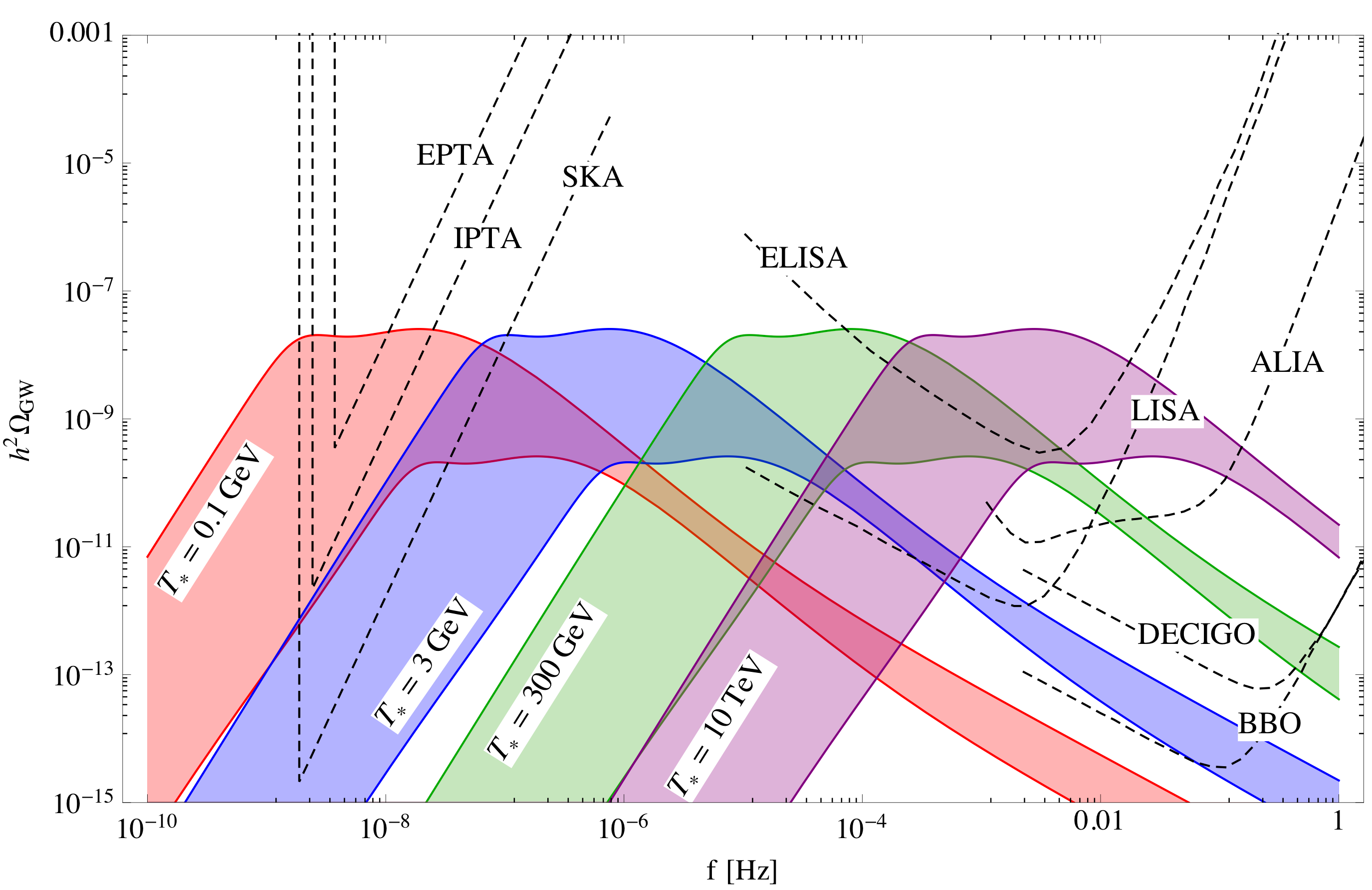
Putting it all together - $h^2 \Omega_\text{gw}$
- For any given theory, can get $T_*$, $\alpha_{T_*}$, $\beta/H_*$, $v_\mathrm{w}$ arXiv:1004.4187
- It's then easy to predict the signal...
(example, $T_* = 94.7~\mathrm{GeV}$, $\alpha_{T_*} =
0.066$, $v_\mathrm{w} =0.95$, $\beta/H_* = 105.9$)
$\mathrm{SNR} = 95$ ☺️
Putting it all together - physical models to GW power spectra
Model ⟶ ($T_*$, $\alpha_{T_*}$, $v_\mathrm{w}$, $\beta$) ⟶ this plot
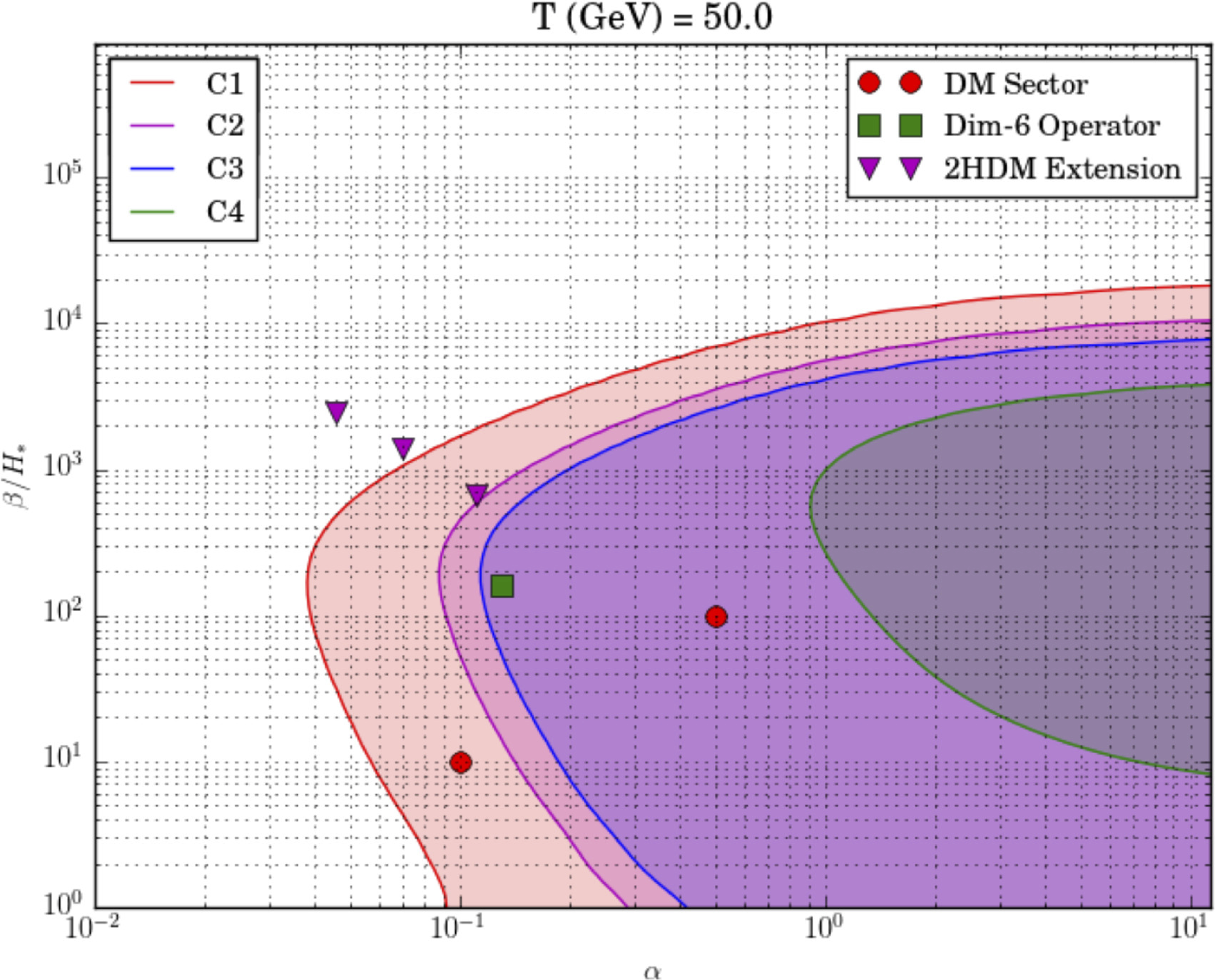
... which tells you if it is detectable by LISA (see arXiv:1512.06239)
PTPlot.org
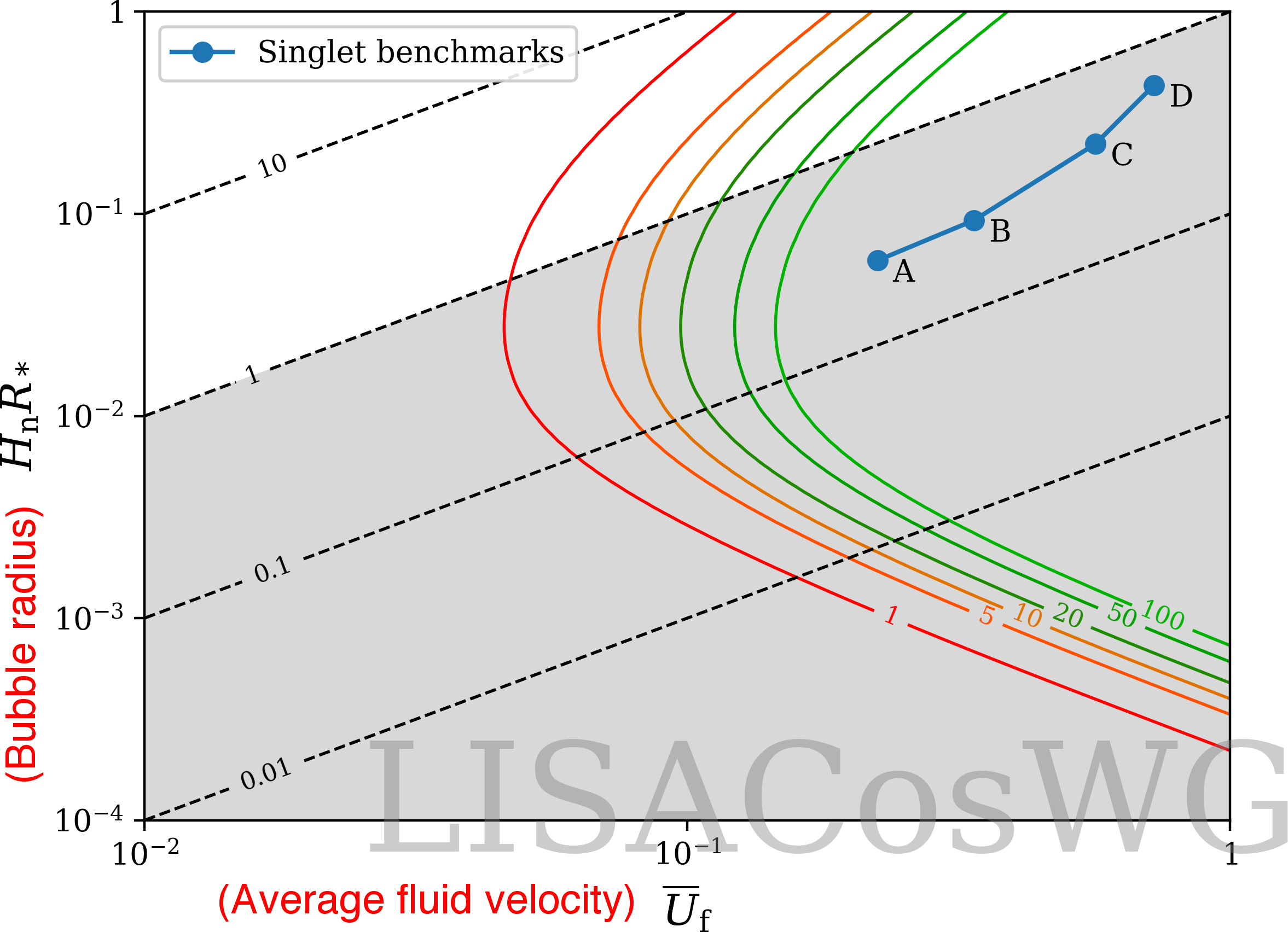
Update this plot?
Final conclusion
- Now have good understanding of thermal history of first-order thermal phase transitions ([near-]vacuum still developing).
- Can make good estimates of the GW power spectrum.
- Turbulence still a challenge.
- Recently appreciated contributions, like acoustic waves, enhance the source considerably.
- LISA provides a model-independent probe of first-order phase transitions around $100~\mathrm{GeV}$.